Home / Hybrid Orbitals and Hybridization
Bonding, Structure, and Resonance
Hybrid Orbitals and Hybridization
Last updated: December 29th, 2024 |
Today’s post is all about hybrid orbitals. Here’s a quick summary:
[Note: This post was co-authored with Matthew Pierce of Organic Chemistry Solutions. Ask Matt about scheduling an online tutoring session here. ]
Table of Contents
- What Orbitals Are Involved In The Tetrahedral Arrangement Of C-H Bonds Around Carbon In Methane (CH4)?
- Hybrid Orbitals: An Explanation For Bonding At Carbon
- A Pop Analogy: Hybrid Soda
- sp3 Hybridization Accurately Describes The Arrangement Of Atoms In (First-Row) Elements Bonded To Four Atoms…
- …As Well As Situations Where The Number of [Atoms + Lone Pairs] Equals 4
- sp2 Hybridization
- sp Hybridization
- Summary – Hybrid Orbitals
- Notes
1. What Orbitals Are Involved In The Tetrahedral Arrangement Of Methane (CH4)?
In the last post on the structure of methane we asked how we know that methane is tetrahedral (See Article: How Do We Know That Methane Is Tetrahedral).
Based on the orbitals of carbon (2s and 2p) we might have naively expected three of the C-H bonds to line up along the x, y, and z axes, respectively, and have the other one at some arbitrary angle (like 125° or so)
But then, as so often happens in science, our beautiful intuitive hypothesis was destroyed by some annoying experimental facts:
- methane has no measurable dipole moment (our “reasonable” structure, below left, would be expected to have a measurable dipole moment)
- the crystal structure of diamond is tetrahedral, with identical bond lengths and angles between carbons of 109.5 degrees. This isn’t what we’d expect if we were dealing with bonds between “pure” 2s and 2p orbitals!
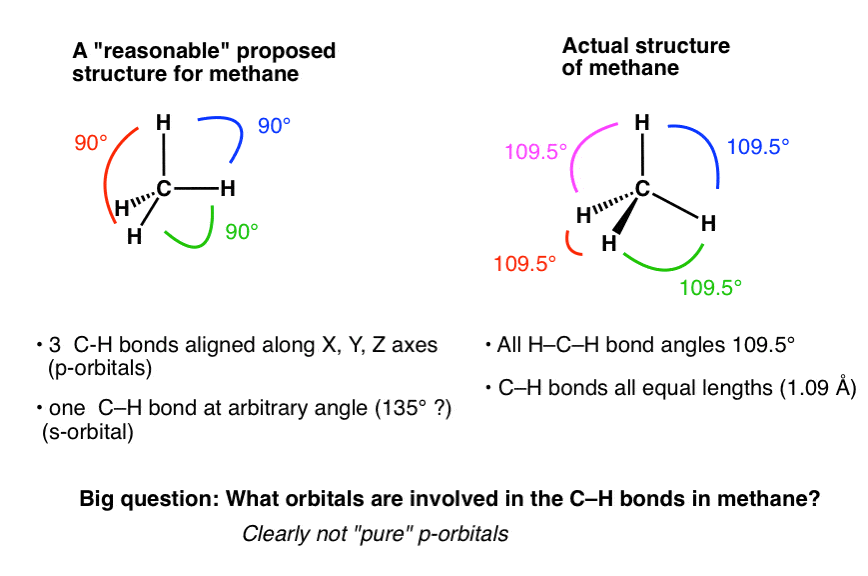
In retrospect, the geometry makes sense. It happens that 109.5 degrees is the orientation that maximizes the distance between each of the four bonding pairs, and thus minimizes their repulsive interactions. In other words, the geometry is a direct consequence of “opposite charges attract, like charges repel“.
But how do we describe the orbitals that are used to give that bond angle?
This is a real chin-scratcher. They can’t be pure 2s orbitals (there’s only one 2s orbital, anyway). And they can’t be pure p orbitals, since the p orbitals are aligned at 90° to each other.
So what the heck kind of orbitals are they?
2. Hybrid Orbitals: An Explanation For Bonding At Carbon
Linus Pauling asked this same question back in his classic treatise, the Nature of the Chemical Bond (1931) [pdf] which in large measure won him the 1954 Nobel Prize in Chemistry.
Pauling’s solution to this dilemma, which we still apply today, was the following:
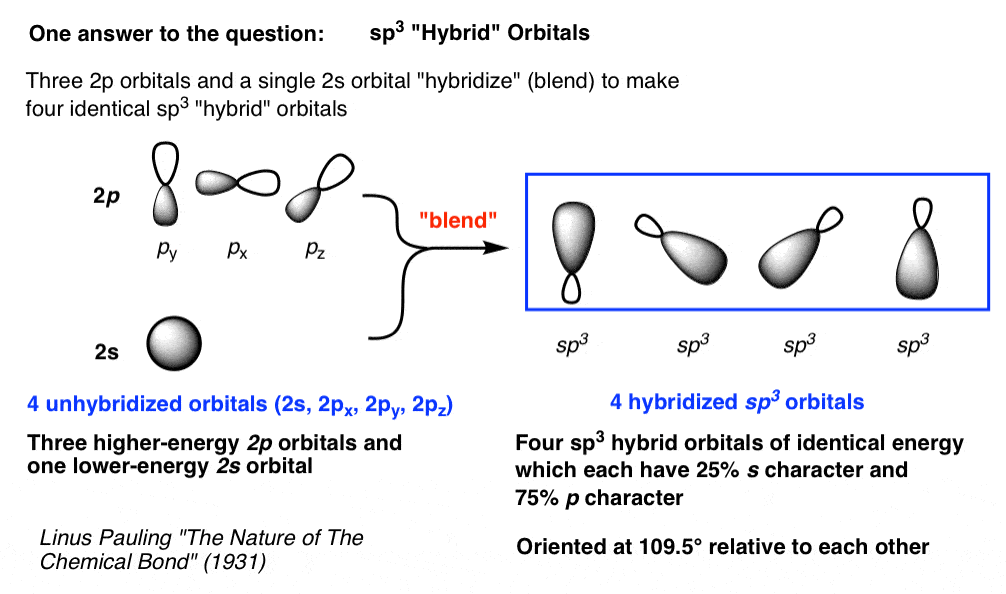
- None of the bonding orbitals in methane are 100% s or 100% p. Instead, they are hybrid orbitals that each have partial s character and partial p character.
- The three 2p orbitals and the single 2s orbital hybridize (i.e., mix) to create four hybrid sp3 orbitals, which are arranged tetrahedrally around the central carbon atom.
- Each of the four hybrid orbitals has 25% s-character and 75% p-character.
- In the case of methane, each of these sp3 hybrid orbitals overlaps with a 1s orbital from hydrogen to form the C-H bonds
I’ll be honest here. Many students don’t like this explanation.
Certainly, it’s confusing at first. Why?
I think that when we initially learn about orbitals, we intuitively think of them as containers – sort of like atomic Tupperware for holding electrons that happen to come in a variety of cutesy shapes.

Electrons, then, can be imagined to behave like the “fruits” that rattle around in these containers (strict limit: 2 per container!). It’s not so surprising to our intuition when we learn that they can move from container to container within an atom or even leave the atom altogether.
What is surprising is when we open up the fridge and find that our cute little sphere- and dumbbell-shaped Tupperware containers have changed their shape and merged their properties with each other! This violates our intuition about how containers behave!
This is the quantum world, folks! If this doesn’t mystify you… it f*&king should!
Going forward, I don’t ask that you understand or even “believe in” hybridization on a deep mathematical or theoretical level. That’s not necessary for our purposes [Pauling was a great teacher. You can always read the original Pauling paper, here, if you choose]
I merely advise that you try to suspend your disbelief going forward, because using this hybridization model will help us rationalize a lot of molecular structure, geometry, and behaviour.
3. A Pop Analogy: Hybrid Soda
Before we dive in any further, here’s what I consider to be a helpful little analogy (thx, Steven) that might help to get the point across.
- Imagine you have four bottles of pop: one bottle of Sprite (S) and three bottles of Pepsi (P).
- Now imagine pouring them out, mixing them all together, and then re-filling each bottle with the mixture.
- The Law of Conservation of Pop says that you still have four bottles worth of liquid. But now the pop is neither pure Sprite or pure Pepsi; it is a hybrid between the two.
- Specifically, each bottle now has 25% Sprite character and 75% Pepsi character.
- We can call this “hybrid” pop, if you like, sp3
![]()
That’s a little bit like what has happened to our 2s and 2p orbitals. By mixing the 2s and three 2p orbitals, we now have four orbitals which have 25% s character and 75% p character. (Although, importantly, it’s the shapes of the bottles that are changing, not just the contents).
The final step is to arrange these four orbitals at the corners of a tetrahedron, which allows for the maximum distance between the four electron pairs (like charges repel!)
How does this rationalization help us?
- It explains the tetrahedral molecular geometry of methane, with 4 identical H–C–H bond angles (109.5°)
- It explains the 4 identical C–H bond lengths (and bond strengths) in methane
- It explains the lack of a dipole moment in methane, since the tetrahedral arrangement of the electron pairs allows for all partial charges to cancel (i.e. the vectors sum to zero)
- The model even helps to rationalize how certain reactions occur, which we won’t go into right now [e.g. backside attack in the SN2 reaction occurs into the empty “antibonding” orbital 180° to the bonding C–H orbital, if you’ve been reading ahead]
4. sp3 Hybridization Accurately Describes The Arrangement Of Atoms In (First-Row) Elements Bonded To Four Atoms…
This doesn’t just apply to methane. It applies to any situation where a (first-row) element is bonded to four atoms. Obviously tetrahedral carbon is the most prominent example we will explore, but it also applies to tetrahedral nitrogen [e.g. NH4(+)] and even tetrahedral boron [e.g. BF4(-)].
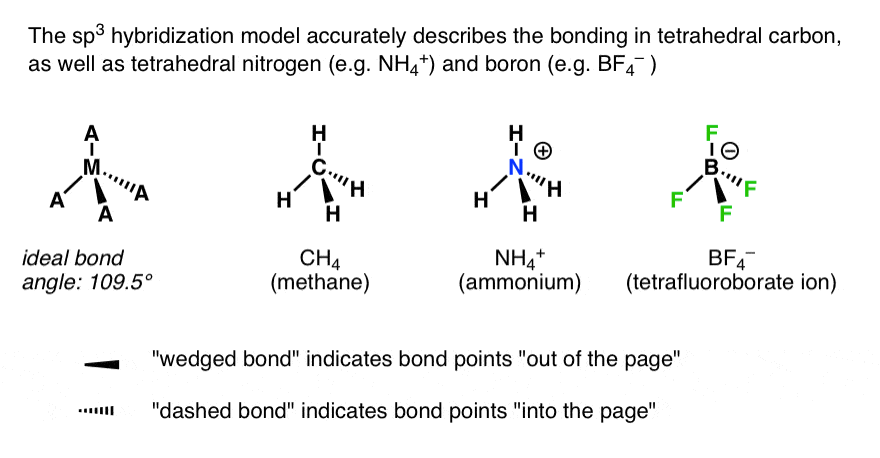
5….As Well As Situations Where The Number of [Atoms + Lone Pairs] Equals 4
The tetrahedral arrangement of the orbitals holds even when one or more pairs of electrons is a non-bonded lone pair (If you’ve run across VSEPR theory, which most people have by the time that they arrive in organic chem, this shouldn’t come as a shock.)
For instance, ammonia (NH3), the “methyl anion” (CH3–) and the hydronium ion (H3O+) all have a central atom with 4 pairs of electrons: 3 pairs of bonding electrons and one pair of non-bonded electrons. As we’ve seen, the ideal geometry for arranging four pairs of electrons is tetrahedral, which makes the hybridization of the central atom sp3. This leaves the molecule with a “piano stool” arrangement of atoms about the central atom, which we call “trigonal pyramidal” geometry.
Another way of putting it is that the central atom has tetrahedral orbital geometry (sp3) and trigonal planar molecular geometry. 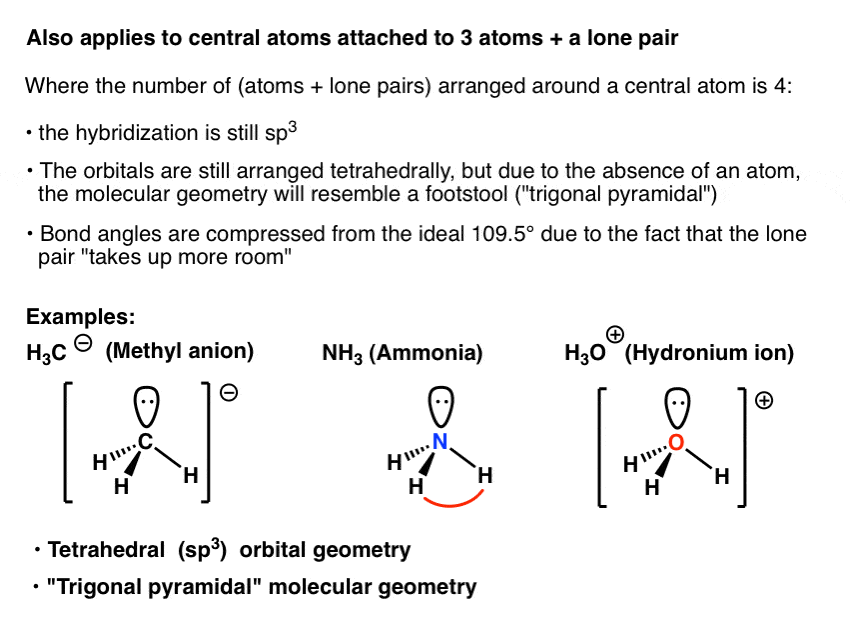
An interesting fact is that the bond angles in these are compressed from the ideal angle of 109.5°. The H-N-H bond angles in ammonia, for instance, are 107 degrees. We rationalize this as being due to the fact that a non-bonded lone pair is more repulsive than a “normal” bonding pair – likely because it’s closer to the atom and exerts a stronger influence.
This deviation from “ideal” bond angles is even greater in H2O (water) which has two lone pairs. The hybridization is still sp3, the orbital geometry is still tetrahedral, but the shape (“molecular geometry”) of the resulting molecule is “bent”.
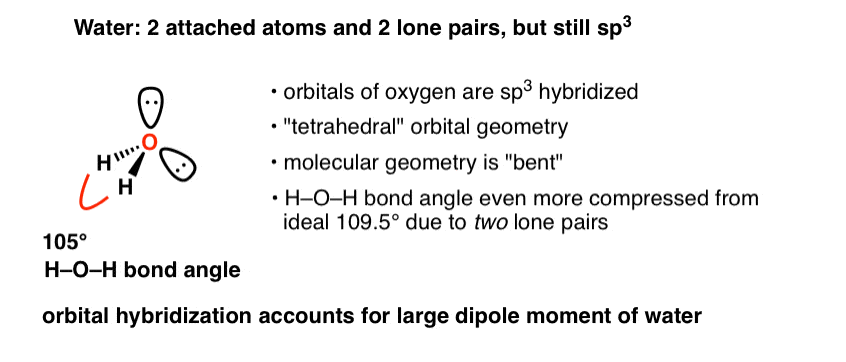
Indeed, one of the notable achievements of Pauling’s hybridization model is that it correctly accounts for the dipole moment of water. If water were perfectly “linear”, as many of us might have naively assumed before we learned any chemistry, the dipoles would cancel each other out.
Another example of “bent” geometry is found in the amide anion NH2– which has two lone pairs on nitrogen.
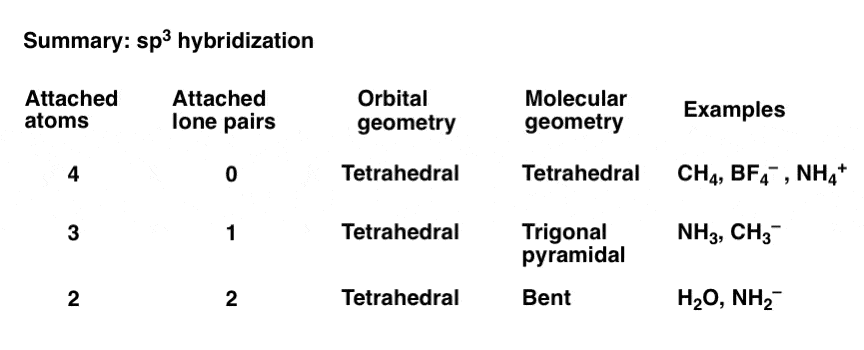
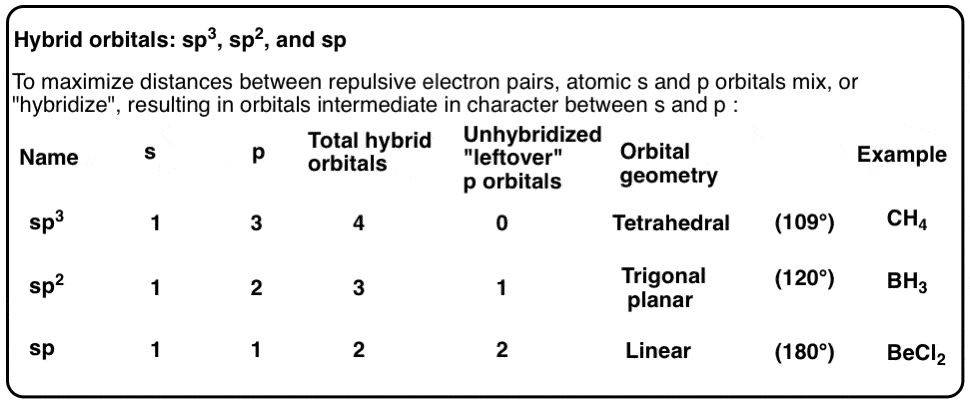
A quick table might help to summarize everything we’ve established about sp3 hybridization:
6. sp2 Hybridization
Let’s go back to our pop-bottle analogy. Say we only mix our Sprite with two bottles of Pepsi, not three.
What does that leave us with?
![]()
This gives us three hybrid bottles of pop, and one leftover unhybridized bottle.
By analogy, if we mix the 2s orbital with two 2p orbitals, we obtain three sp2 hybrid orbitals, with one unhybridized p-orbital left over.
When these three sp2 orbitals are filled with electron pairs, the bond angle that maximizes their distance apart is 120°.
This gives us a “trigonal planar” arrangement of sp2 orbitals, with the unhybridized p orbital at right angles to the plane. It kind of resembles a Mercedes Benz symbol.
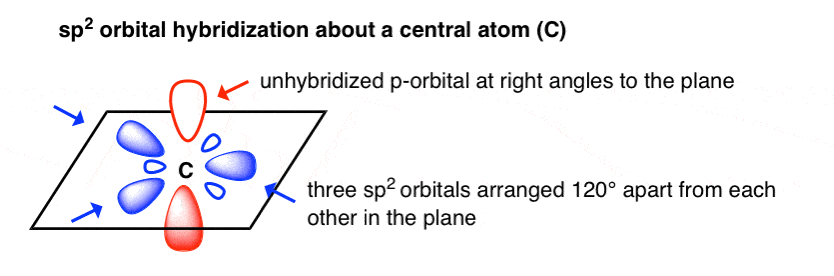
A classic example of this trigonal planar geometry is seen in borane, BH3 , which has three pairs of bonding electrons arranged at 120° to each other. The trigonal planar geometry is also found in carbocations, such as the methyl cation, CH3+ .

You might wonder: where is the unhybridized p orbital?
At right angles to the plane. Recall that each of the three p orbitals are at right angles to each other. So whichever two p-orbitals hybridize, the third (leftover) p orbital will be at right angles to the plane that they form (just like the z axis is perpendicular to the xy plane)
In the case of BH3 and carbocations, the unhybridized p-orbital is empty.
There is another very common situation where sp2 geometry is observed, however. If adjacent atoms have single electrons in unhybridized p-orbitals, and if those p-orbitals can overlap, a bond can result. This is a phenomenon known as “pi-bonding“. (We’ll have a lot more to say about it later.)
Pi bonds – which are often just called, “double bonds” – require an unhybridized p orbital in order to form.
The carbon, oxygen, and nitrogen atoms in the examples below, which all have pi bonds (double bonds) are sp2 hybridized. The orbitals are separated by angles of approximately 120°.
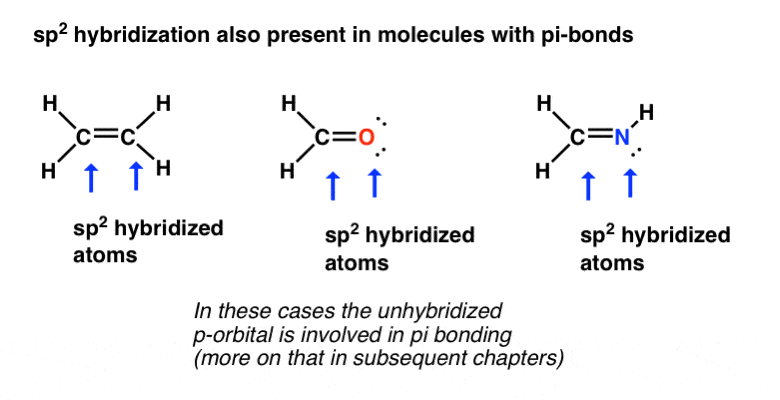
Note that lone pairs can be in sp2-hybridized orbitals, just as we saw in NH3 and H2O in the case of sp3 hybridization. Also note that in the midde molecule (formaldehyde), the oxygen has two lone pairs (each in sp2 hybridized orbitals) and in the top right molecule the nitrogen has a single lone pair in a sp2 hybridized orbital.
When a lone pair is present, the bond angles will be slightly less than 120° since the lone pair can be thought of taking up more “room”.
7. sp Hybridization
Let’s examine the last possible case. What if only one p orbital hybridizes with the s orbital?
This gives us two hybrid “sp” orbitals separated by the maximum angle apart: 180 degrees. We call this arrangement, “linear”. Each hybrid sp orbital has 50% s character and 50% p character.
![]()
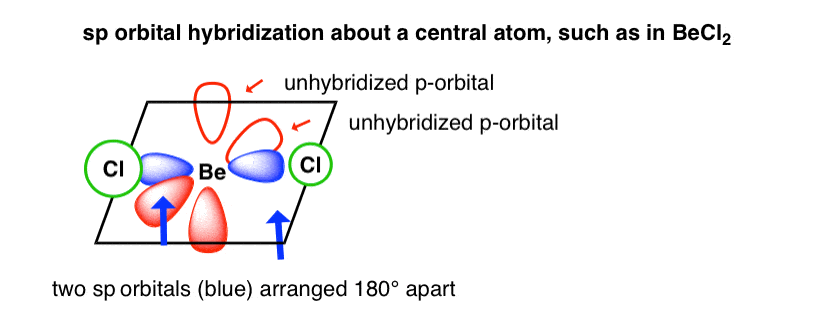
The two unhybridized p-orbitals are each at right angles to the sp hybrid orbitals.
For instance, here’s what the orbitals look like in beryllium chloride (BeCl2) where the Cl-Be-Cl bond angle is 180°. If we consider the Cl-Be-Cl bond to be along the x-axis, the two (unhybridized) p-orbitals will be along the y and z axes, respectively.
sp-hybridization is more commonly observed in situations where there are two pi bonds on a single atom. The most prominent examples are “triple bonds”, as seen in alkynes, nitriles, and carbon monoxide (CO). In these cases, not only are the carbon atoms sp-hybridized, but so are the nitrogen (in nitriles) and oxygen (in carbon monoxide) atoms.
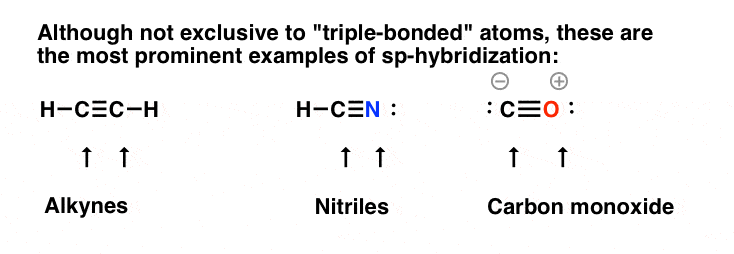
Note also that lone pairs can be in sp-hybridized orbitals, as seen in nitriles and carbon monoxide.
sp-hybridization is not exclusive to triple bonded atoms – for example, the central carbons in allene and ketene participate in two pi bonds and are therefore sp-hybridized – but triple bonds are the most immediately recognizable examples.
8. Summary – Hybrid Orbitals
OK. Here are the main points of this unintentionally very long post:
- Electron pairs repel. In the absence of orbital hybridization, the bond angles around CH4 would be confined to the geometry of the p-orbitals (90°). It’s energetically favorable for the s and p orbitals to hybridize to form sp3 orbitals which results in a greater separation of the electron pairs and bond angles of 109° (i.e. at the apices of a tetrahedron). This also holds for central atoms with non-bonding electron pairs such as NH3 and H2O.
- When only two p orbitals participate in hybridization, three sp2 hybrid orbitals result that adopt a trigonal planar orbital geometry. The remaining (unhybridized) p orbital, which is at right angles to the trigonal plane, can either be empty (as in BH3) or singly-occupied (as seen in molecules containing pi bonds).
- When only one p orbital participates in hybridization, the result is two sp hybrid orbitals and a linear orbital geometry. The two remaining p orbitals are available for pi-bonding (as in triply-bonded organic compounds such as alkynes and nitriles) or can be empty (as in BeCl2).
- Note that the number of orbitals around the central atom is always 4. Orbitals are neither created nor destroyed by hybridization; they are merely transformed.
In the next post we will just provide a super simple trick for quickly determining the hybridization of a central atom.
Thanks again to Matt for co-authoring. Ask Matt about scheduling an online tutoring session here.
Notes
Related Articles
- How To Determine Hybridization: A Shortcut
- How Do We Know Methane (CH4) Is Tetrahedral?
- Valence Electrons of the First Row Elements
- A Key Skill: How to Calculate Formal Charge
- Introduction to Resonance
- Conjugation And Resonance In Organic Chemistry
- In Summary: Evaluating Resonance Structures
- Bond Hybridization Practice (MOC Membership)
- Structure and Bonding Practice Quizzes (MOC Membership)
00 General Chemistry Review
01 Bonding, Structure, and Resonance
- How Do We Know Methane (CH4) Is Tetrahedral?
- Hybrid Orbitals and Hybridization
- How To Determine Hybridization: A Shortcut
- Orbital Hybridization And Bond Strengths
- Sigma bonds come in six varieties: Pi bonds come in one
- A Key Skill: How to Calculate Formal Charge
- The Four Intermolecular Forces and How They Affect Boiling Points
- 3 Trends That Affect Boiling Points
- How To Use Electronegativity To Determine Electron Density (and why NOT to trust formal charge)
- Introduction to Resonance
- How To Use Curved Arrows To Interchange Resonance Forms
- Evaluating Resonance Forms (1) - The Rule of Least Charges
- How To Find The Best Resonance Structure By Applying Electronegativity
- Evaluating Resonance Structures With Negative Charges
- Evaluating Resonance Structures With Positive Charge
- Exploring Resonance: Pi-Donation
- Exploring Resonance: Pi-acceptors
- In Summary: Evaluating Resonance Structures
- Drawing Resonance Structures: 3 Common Mistakes To Avoid
- How to apply electronegativity and resonance to understand reactivity
- Bond Hybridization Practice
- Structure and Bonding Practice Quizzes
- Resonance Structures Practice
02 Acid Base Reactions
- Introduction to Acid-Base Reactions
- Acid Base Reactions In Organic Chemistry
- The Stronger The Acid, The Weaker The Conjugate Base
- Walkthrough of Acid-Base Reactions (3) - Acidity Trends
- Five Key Factors That Influence Acidity
- Acid-Base Reactions: Introducing Ka and pKa
- How to Use a pKa Table
- The pKa Table Is Your Friend
- A Handy Rule of Thumb for Acid-Base Reactions
- Acid Base Reactions Are Fast
- pKa Values Span 60 Orders Of Magnitude
- How Protonation and Deprotonation Affect Reactivity
- Acid Base Practice Problems
03 Alkanes and Nomenclature
- Meet the (Most Important) Functional Groups
- Condensed Formulas: Deciphering What the Brackets Mean
- Hidden Hydrogens, Hidden Lone Pairs, Hidden Counterions
- Don't Be Futyl, Learn The Butyls
- Primary, Secondary, Tertiary, Quaternary In Organic Chemistry
- Branching, and Its Affect On Melting and Boiling Points
- The Many, Many Ways of Drawing Butane
- Wedge And Dash Convention For Tetrahedral Carbon
- Common Mistakes in Organic Chemistry: Pentavalent Carbon
- Table of Functional Group Priorities for Nomenclature
- Summary Sheet - Alkane Nomenclature
- Organic Chemistry IUPAC Nomenclature Demystified With A Simple Puzzle Piece Approach
- Boiling Point Quizzes
- Organic Chemistry Nomenclature Quizzes
04 Conformations and Cycloalkanes
- Staggered vs Eclipsed Conformations of Ethane
- Conformational Isomers of Propane
- Newman Projection of Butane (and Gauche Conformation)
- Introduction to Cycloalkanes
- Geometric Isomers In Small Rings: Cis And Trans Cycloalkanes
- Calculation of Ring Strain In Cycloalkanes
- Cycloalkanes - Ring Strain In Cyclopropane And Cyclobutane
- Cyclohexane Conformations
- Cyclohexane Chair Conformation: An Aerial Tour
- How To Draw The Cyclohexane Chair Conformation
- The Cyclohexane Chair Flip
- The Cyclohexane Chair Flip - Energy Diagram
- Substituted Cyclohexanes - Axial vs Equatorial
- Ranking The Bulkiness Of Substituents On Cyclohexanes: "A-Values"
- Cyclohexane Chair Conformation Stability: Which One Is Lower Energy?
- Fused Rings - Cis-Decalin and Trans-Decalin
- Naming Bicyclic Compounds - Fused, Bridged, and Spiro
- Bredt's Rule (And Summary of Cycloalkanes)
- Newman Projection Practice
- Cycloalkanes Practice Problems
05 A Primer On Organic Reactions
- The Most Important Question To Ask When Learning a New Reaction
- Curved Arrows (for reactions)
- Nucleophiles and Electrophiles
- The Three Classes of Nucleophiles
- Nucleophilicity vs. Basicity
- What Makes A Good Nucleophile?
- What Makes A Good Leaving Group?
- 3 Factors That Stabilize Carbocations
- Equilibrium and Energy Relationships
- 7 Factors that stabilize negative charge in organic chemistry
- 7 Factors That Stabilize Positive Charge in Organic Chemistry
- What's a Transition State?
- Hammond's Postulate
- Learning Organic Chemistry Reactions: A Checklist (PDF)
- Introduction to Oxidative Cleavage Reactions
06 Free Radical Reactions
- Free Radical Reactions
- 3 Factors That Stabilize Free Radicals
- Bond Strengths And Radical Stability
- Free Radical Initiation: Why Is "Light" Or "Heat" Required?
- Initiation, Propagation, Termination
- Monochlorination Products Of Propane, Pentane, And Other Alkanes
- Selectivity In Free Radical Reactions
- Selectivity in Free Radical Reactions: Bromination vs. Chlorination
- Halogenation At Tiffany's
- Allylic Bromination
- Bonus Topic: Allylic Rearrangements
- In Summary: Free Radicals
- Synthesis (2) - Reactions of Alkanes
- Free Radicals Practice Quizzes
07 Stereochemistry and Chirality
- Types of Isomers: Constitutional Isomers, Stereoisomers, Enantiomers, and Diastereomers
- How To Draw The Enantiomer Of A Chiral Molecule
- How To Draw A Bond Rotation
- Introduction to Assigning (R) and (S): The Cahn-Ingold-Prelog Rules
- Assigning Cahn-Ingold-Prelog (CIP) Priorities (2) - The Method of Dots
- Enantiomers vs Diastereomers vs The Same? Two Methods For Solving Problems
- Assigning R/S To Newman Projections (And Converting Newman To Line Diagrams)
- How To Determine R and S Configurations On A Fischer Projection
- The Meso Trap
- Optical Rotation, Optical Activity, and Specific Rotation
- Optical Purity and Enantiomeric Excess
- What's a Racemic Mixture?
- Chiral Allenes And Chiral Axes
- Stereochemistry Practice Problems and Quizzes
08 Substitution Reactions
- Nucleophilic Substitution Reactions - Introduction
- Two Types of Nucleophilic Substitution Reactions
- The SN2 Mechanism
- Why the SN2 Reaction Is Powerful
- The SN1 Mechanism
- The Conjugate Acid Is A Better Leaving Group
- Comparing the SN1 and SN2 Reactions
- Polar Protic? Polar Aprotic? Nonpolar? All About Solvents
- Steric Hindrance is Like a Fat Goalie
- Common Blind Spot: Intramolecular Reactions
- Substitution Practice - SN1
- Substitution Practice - SN2
09 Elimination Reactions
- Elimination Reactions (1): Introduction And The Key Pattern
- Elimination Reactions (2): The Zaitsev Rule
- Elimination Reactions Are Favored By Heat
- Two Elimination Reaction Patterns
- The E1 Reaction
- The E2 Mechanism
- E1 vs E2: Comparing the E1 and E2 Reactions
- Antiperiplanar Relationships: The E2 Reaction and Cyclohexane Rings
- Bulky Bases in Elimination Reactions
- Comparing the E1 vs SN1 Reactions
- Elimination (E1) Reactions With Rearrangements
- E1cB - Elimination (Unimolecular) Conjugate Base
- Elimination (E1) Practice Problems And Solutions
- Elimination (E2) Practice Problems and Solutions
10 Rearrangements
11 SN1/SN2/E1/E2 Decision
- Identifying Where Substitution and Elimination Reactions Happen
- Deciding SN1/SN2/E1/E2 (1) - The Substrate
- Deciding SN1/SN2/E1/E2 (2) - The Nucleophile/Base
- SN1 vs E1 and SN2 vs E2 : The Temperature
- Deciding SN1/SN2/E1/E2 - The Solvent
- Wrapup: The Key Factors For Determining SN1/SN2/E1/E2
- Alkyl Halide Reaction Map And Summary
- SN1 SN2 E1 E2 Practice Problems
12 Alkene Reactions
- E and Z Notation For Alkenes (+ Cis/Trans)
- Alkene Stability
- Alkene Addition Reactions: "Regioselectivity" and "Stereoselectivity" (Syn/Anti)
- Stereoselective and Stereospecific Reactions
- Hydrohalogenation of Alkenes and Markovnikov's Rule
- Hydration of Alkenes With Aqueous Acid
- Rearrangements in Alkene Addition Reactions
- Halogenation of Alkenes and Halohydrin Formation
- Oxymercuration Demercuration of Alkenes
- Hydroboration Oxidation of Alkenes
- m-CPBA (meta-chloroperoxybenzoic acid)
- OsO4 (Osmium Tetroxide) for Dihydroxylation of Alkenes
- Palladium on Carbon (Pd/C) for Catalytic Hydrogenation of Alkenes
- Cyclopropanation of Alkenes
- A Fourth Alkene Addition Pattern - Free Radical Addition
- Alkene Reactions: Ozonolysis
- Summary: Three Key Families Of Alkene Reaction Mechanisms
- Synthesis (4) - Alkene Reaction Map, Including Alkyl Halide Reactions
- Alkene Reactions Practice Problems
13 Alkyne Reactions
- Acetylides from Alkynes, And Substitution Reactions of Acetylides
- Partial Reduction of Alkynes With Lindlar's Catalyst
- Partial Reduction of Alkynes With Na/NH3 To Obtain Trans Alkenes
- Alkyne Hydroboration With "R2BH"
- Hydration and Oxymercuration of Alkynes
- Hydrohalogenation of Alkynes
- Alkyne Halogenation: Bromination and Chlorination of Alkynes
- Oxidation of Alkynes With O3 and KMnO4
- Alkenes To Alkynes Via Halogenation And Elimination Reactions
- Alkynes Are A Blank Canvas
- Synthesis (5) - Reactions of Alkynes
- Alkyne Reactions Practice Problems With Answers
14 Alcohols, Epoxides and Ethers
- Alcohols - Nomenclature and Properties
- Alcohols Can Act As Acids Or Bases (And Why It Matters)
- Alcohols - Acidity and Basicity
- The Williamson Ether Synthesis
- Ethers From Alkenes, Tertiary Alkyl Halides and Alkoxymercuration
- Alcohols To Ethers via Acid Catalysis
- Cleavage Of Ethers With Acid
- Epoxides - The Outlier Of The Ether Family
- Opening of Epoxides With Acid
- Epoxide Ring Opening With Base
- Making Alkyl Halides From Alcohols
- Tosylates And Mesylates
- PBr3 and SOCl2
- Elimination Reactions of Alcohols
- Elimination of Alcohols To Alkenes With POCl3
- Alcohol Oxidation: "Strong" and "Weak" Oxidants
- Demystifying The Mechanisms of Alcohol Oxidations
- Protecting Groups For Alcohols
- Thiols And Thioethers
- Calculating the oxidation state of a carbon
- Oxidation and Reduction in Organic Chemistry
- Oxidation Ladders
- SOCl2 Mechanism For Alcohols To Alkyl Halides: SN2 versus SNi
- Alcohol Reactions Roadmap (PDF)
- Alcohol Reaction Practice Problems
- Epoxide Reaction Quizzes
- Oxidation and Reduction Practice Quizzes
15 Organometallics
- What's An Organometallic?
- Formation of Grignard and Organolithium Reagents
- Organometallics Are Strong Bases
- Reactions of Grignard Reagents
- Protecting Groups In Grignard Reactions
- Synthesis Problems Involving Grignard Reagents
- Grignard Reactions And Synthesis (2)
- Organocuprates (Gilman Reagents): How They're Made
- Gilman Reagents (Organocuprates): What They're Used For
- The Heck, Suzuki, and Olefin Metathesis Reactions (And Why They Don't Belong In Most Introductory Organic Chemistry Courses)
- Reaction Map: Reactions of Organometallics
- Grignard Practice Problems
16 Spectroscopy
- Degrees of Unsaturation (or IHD, Index of Hydrogen Deficiency)
- Conjugation And Color (+ How Bleach Works)
- Introduction To UV-Vis Spectroscopy
- UV-Vis Spectroscopy: Absorbance of Carbonyls
- UV-Vis Spectroscopy: Practice Questions
- Bond Vibrations, Infrared Spectroscopy, and the "Ball and Spring" Model
- Infrared Spectroscopy: A Quick Primer On Interpreting Spectra
- IR Spectroscopy: 4 Practice Problems
- 1H NMR: How Many Signals?
- Homotopic, Enantiotopic, Diastereotopic
- Diastereotopic Protons in 1H NMR Spectroscopy: Examples
- 13-C NMR - How Many Signals
- Liquid Gold: Pheromones In Doe Urine
- Natural Product Isolation (1) - Extraction
- Natural Product Isolation (2) - Purification Techniques, An Overview
- Structure Determination Case Study: Deer Tarsal Gland Pheromone
17 Dienes and MO Theory
- What To Expect In Organic Chemistry 2
- Are these molecules conjugated?
- Conjugation And Resonance In Organic Chemistry
- Bonding And Antibonding Pi Orbitals
- Molecular Orbitals of The Allyl Cation, Allyl Radical, and Allyl Anion
- Pi Molecular Orbitals of Butadiene
- Reactions of Dienes: 1,2 and 1,4 Addition
- Thermodynamic and Kinetic Products
- More On 1,2 and 1,4 Additions To Dienes
- s-cis and s-trans
- The Diels-Alder Reaction
- Cyclic Dienes and Dienophiles in the Diels-Alder Reaction
- Stereochemistry of the Diels-Alder Reaction
- Exo vs Endo Products In The Diels Alder: How To Tell Them Apart
- HOMO and LUMO In the Diels Alder Reaction
- Why Are Endo vs Exo Products Favored in the Diels-Alder Reaction?
- Diels-Alder Reaction: Kinetic and Thermodynamic Control
- The Retro Diels-Alder Reaction
- The Intramolecular Diels Alder Reaction
- Regiochemistry In The Diels-Alder Reaction
- The Cope and Claisen Rearrangements
- Electrocyclic Reactions
- Electrocyclic Ring Opening And Closure (2) - Six (or Eight) Pi Electrons
- Diels Alder Practice Problems
- Molecular Orbital Theory Practice
18 Aromaticity
- Introduction To Aromaticity
- Rules For Aromaticity
- Huckel's Rule: What Does 4n+2 Mean?
- Aromatic, Non-Aromatic, or Antiaromatic? Some Practice Problems
- Antiaromatic Compounds and Antiaromaticity
- The Pi Molecular Orbitals of Benzene
- The Pi Molecular Orbitals of Cyclobutadiene
- Frost Circles
- Aromaticity Practice Quizzes
19 Reactions of Aromatic Molecules
- Electrophilic Aromatic Substitution: Introduction
- Activating and Deactivating Groups In Electrophilic Aromatic Substitution
- Electrophilic Aromatic Substitution - The Mechanism
- Ortho-, Para- and Meta- Directors in Electrophilic Aromatic Substitution
- Understanding Ortho, Para, and Meta Directors
- Why are halogens ortho- para- directors?
- Disubstituted Benzenes: The Strongest Electron-Donor "Wins"
- Electrophilic Aromatic Substitutions (1) - Halogenation of Benzene
- Electrophilic Aromatic Substitutions (2) - Nitration and Sulfonation
- EAS Reactions (3) - Friedel-Crafts Acylation and Friedel-Crafts Alkylation
- Intramolecular Friedel-Crafts Reactions
- Nucleophilic Aromatic Substitution (NAS)
- Nucleophilic Aromatic Substitution (2) - The Benzyne Mechanism
- Reactions on the "Benzylic" Carbon: Bromination And Oxidation
- The Wolff-Kishner, Clemmensen, And Other Carbonyl Reductions
- More Reactions on the Aromatic Sidechain: Reduction of Nitro Groups and the Baeyer Villiger
- Aromatic Synthesis (1) - "Order Of Operations"
- Synthesis of Benzene Derivatives (2) - Polarity Reversal
- Aromatic Synthesis (3) - Sulfonyl Blocking Groups
- Birch Reduction
- Synthesis (7): Reaction Map of Benzene and Related Aromatic Compounds
- Aromatic Reactions and Synthesis Practice
- Electrophilic Aromatic Substitution Practice Problems
20 Aldehydes and Ketones
- What's The Alpha Carbon In Carbonyl Compounds?
- Nucleophilic Addition To Carbonyls
- Aldehydes and Ketones: 14 Reactions With The Same Mechanism
- Sodium Borohydride (NaBH4) Reduction of Aldehydes and Ketones
- Grignard Reagents For Addition To Aldehydes and Ketones
- Wittig Reaction
- Hydrates, Hemiacetals, and Acetals
- Imines - Properties, Formation, Reactions, and Mechanisms
- All About Enamines
- Breaking Down Carbonyl Reaction Mechanisms: Reactions of Anionic Nucleophiles (Part 2)
- Aldehydes Ketones Reaction Practice
21 Carboxylic Acid Derivatives
- Nucleophilic Acyl Substitution (With Negatively Charged Nucleophiles)
- Addition-Elimination Mechanisms With Neutral Nucleophiles (Including Acid Catalysis)
- Basic Hydrolysis of Esters - Saponification
- Transesterification
- Proton Transfer
- Fischer Esterification - Carboxylic Acid to Ester Under Acidic Conditions
- Lithium Aluminum Hydride (LiAlH4) For Reduction of Carboxylic Acid Derivatives
- LiAlH[Ot-Bu]3 For The Reduction of Acid Halides To Aldehydes
- Di-isobutyl Aluminum Hydride (DIBAL) For The Partial Reduction of Esters and Nitriles
- Amide Hydrolysis
- Thionyl Chloride (SOCl2) And Conversion of Carboxylic Acids to Acid Halides
- Diazomethane (CH2N2)
- Carbonyl Chemistry: Learn Six Mechanisms For the Price Of One
- Making Music With Mechanisms (PADPED)
- Carboxylic Acid Derivatives Practice Questions
22 Enols and Enolates
- Keto-Enol Tautomerism
- Enolates - Formation, Stability, and Simple Reactions
- Kinetic Versus Thermodynamic Enolates
- Aldol Addition and Condensation Reactions
- Reactions of Enols - Acid-Catalyzed Aldol, Halogenation, and Mannich Reactions
- Claisen Condensation and Dieckmann Condensation
- Decarboxylation
- The Malonic Ester and Acetoacetic Ester Synthesis
- The Michael Addition Reaction and Conjugate Addition
- The Robinson Annulation
- Haloform Reaction
- The Hell–Volhard–Zelinsky Reaction
- Enols and Enolates Practice Quizzes
23 Amines
- The Amide Functional Group: Properties, Synthesis, and Nomenclature
- Basicity of Amines And pKaH
- 5 Key Basicity Trends of Amines
- The Mesomeric Effect And Aromatic Amines
- Nucleophilicity of Amines
- Alkylation of Amines (Sucks!)
- Reductive Amination
- The Gabriel Synthesis
- Some Reactions of Azides
- The Hofmann Elimination
- The Hofmann and Curtius Rearrangements
- The Cope Elimination
- Protecting Groups for Amines - Carbamates
- The Strecker Synthesis of Amino Acids
- Introduction to Peptide Synthesis
- Reactions of Diazonium Salts: Sandmeyer and Related Reactions
- Amine Practice Questions
24 Carbohydrates
- D and L Notation For Sugars
- Pyranoses and Furanoses: Ring-Chain Tautomerism In Sugars
- What is Mutarotation?
- Reducing Sugars
- The Big Damn Post Of Carbohydrate-Related Chemistry Definitions
- The Haworth Projection
- Converting a Fischer Projection To A Haworth (And Vice Versa)
- Reactions of Sugars: Glycosylation and Protection
- The Ruff Degradation and Kiliani-Fischer Synthesis
- Isoelectric Points of Amino Acids (and How To Calculate Them)
- Carbohydrates Practice
- Amino Acid Quizzes
25 Fun and Miscellaneous
- A Gallery of Some Interesting Molecules From Nature
- Screw Organic Chemistry, I'm Just Going To Write About Cats
- On Cats, Part 1: Conformations and Configurations
- On Cats, Part 2: Cat Line Diagrams
- On Cats, Part 4: Enantiocats
- On Cats, Part 6: Stereocenters
- Organic Chemistry Is Shit
- The Organic Chemistry Behind "The Pill"
- Maybe they should call them, "Formal Wins" ?
- Why Do Organic Chemists Use Kilocalories?
- The Principle of Least Effort
- Organic Chemistry GIFS - Resonance Forms
- Reproducibility In Organic Chemistry
- What Holds The Nucleus Together?
- How Reactions Are Like Music
- Organic Chemistry and the New MCAT
26 Organic Chemistry Tips and Tricks
- Common Mistakes: Formal Charges Can Mislead
- Partial Charges Give Clues About Electron Flow
- Draw The Ugly Version First
- Organic Chemistry Study Tips: Learn the Trends
- The 8 Types of Arrows In Organic Chemistry, Explained
- Top 10 Skills To Master Before An Organic Chemistry 2 Final
- Common Mistakes with Carbonyls: Carboxylic Acids... Are Acids!
- Planning Organic Synthesis With "Reaction Maps"
- Alkene Addition Pattern #1: The "Carbocation Pathway"
- Alkene Addition Pattern #2: The "Three-Membered Ring" Pathway
- Alkene Addition Pattern #3: The "Concerted" Pathway
- Number Your Carbons!
- The 4 Major Classes of Reactions in Org 1
- How (and why) electrons flow
- Grossman's Rule
- Three Exam Tips
- A 3-Step Method For Thinking Through Synthesis Problems
- Putting It Together
- Putting Diels-Alder Products in Perspective
- The Ups and Downs of Cyclohexanes
- The Most Annoying Exceptions in Org 1 (Part 1)
- The Most Annoying Exceptions in Org 1 (Part 2)
- The Marriage May Be Bad, But the Divorce Still Costs Money
- 9 Nomenclature Conventions To Know
- Nucleophile attacks Electrophile
27 Case Studies of Successful O-Chem Students
- Success Stories: How Corina Got The The "Hard" Professor - And Got An A+ Anyway
- How Helena Aced Organic Chemistry
- From a "Drop" To B+ in Org 2 – How A Hard Working Student Turned It Around
- How Serge Aced Organic Chemistry
- Success Stories: How Zach Aced Organic Chemistry 1
- Success Stories: How Kari Went From C– to B+
- How Esther Bounced Back From a "C" To Get A's In Organic Chemistry 1 And 2
- How Tyrell Got The Highest Grade In Her Organic Chemistry Course
- This Is Why Students Use Flashcards
- Success Stories: How Stu Aced Organic Chemistry
- How John Pulled Up His Organic Chemistry Exam Grades
- Success Stories: How Nathan Aced Organic Chemistry (Without It Taking Over His Life)
- How Chris Aced Org 1 and Org 2
- Interview: How Jay Got an A+ In Organic Chemistry
- How to Do Well in Organic Chemistry: One Student's Advice
- "America's Top TA" Shares His Secrets For Teaching O-Chem
- "Organic Chemistry Is Like..." - A Few Metaphors
- How To Do Well In Organic Chemistry: Advice From A Tutor
- Guest post: "I went from being afraid of tests to actually looking forward to them".
Thank you for great explanation.
I was wondering what determines how many p orbitals used for hybridization. For example, CH4 use 3 three p orbitals for hybridization, while BH3 use only two.
Thank you
The number of [single bonds + lone pairs] on the central atom
– 4 [single bonds + lone pairs] —-> sp3 hybridization – one s orbital, 3 p orbitals
– 3 [single bonds + lone pairs] —-> sp2 hybridization – one s orbital, 2 p orbitals
– 2 [single bonds + lone pairs] =—> sp hybridization – one s orbital, 1 p orbital.
CH4 has a total of 4 single bonds around the central atom and zero lone pairs. sp3 hybridization – one s orbital, 3 p orbitals
BH3 has a total of 3 single bonds around the central atom and zero lone pairs. sp2 hybridization – one s orbital, 2 p orbitals
The present work shows the inapplicability of the Pauli principle to chemical bond, and a new theoretical model of the chemical bond is proposed based on the Heisenberg uncertainty principle.
See pp. 88 – 104 Review. Benzene on the Basis of the Three-Electron Bond. (The Pauli exclusion principle, Heisenberg’s uncertainty principle and chemical bond). http://vixra.org/pdf/1710.0326v1.pdf
The Pauli exclusion principle and the chemical bond.
The Pauli exclusion principle — this is the fundamental principle of quantum mechanics, which asserts that two or more identical fermions (particles with half-integral spin) can not simultaneously be in the same quantum state.
Wolfgang Pauli, a Swiss theoretical physicist, formulated this principle in 1925 [1]. In chemistry exactly Pauli exclusion principle often considered as a ban on the existence of three-electron bonds with a multiplicity of 1.5, but it can be shown that Pauli exclusion principle does not prohibit the existence of three-electron bonds. To do this, analyze the Pauli exclusion principle in more detail.
According to Pauli exclusion principle in a system consisting of identical fermions, two (or more) particles can not be in the same states [2]. The corresponding formulas of the wave functions and the determinant are given in the reference (this is a standard consideration of the fermion system), but we will concentrate our attention on the derivation: “… Of course, in this formulation, Pauli exclusion principle can only be applied to systems of weakly interacting particles, when one can speak (at least approximately on the states of individual particles) “[2]. That is, Pauli exclusion principle can only be applied to weakly interacting particles, when one can talk about the states of individual particles.
But if we recall that any classical chemical bond is formed between two nuclei (this is a fundamental difference from atomic orbitals), which somehow “pull” the electrons one upon another, it is logical to assume that in the formation of a chemical bond, the electrons can no longer be regarded as weakly interacting particles . This assumption is confirmed by the earlier introduced notion of a chemical bond as a separate semi-virtual particle (natural component of the particle “parts” can not be weakly interacting).
Representations of the chemical bond given in the chapter “The Principle of Heisenberg’s Uncertainty and the Chemical Bond” categorically reject the statements about the chemical bond as a system of weakly interacting electrons. On the contrary, it follows from the above description that in the chemical bond, the electrons “lose” their individuality and “occupy” the entire chemical bond, that is, the electrons in the chemical bond “interact as much as possible”, which directly indicates the inapplicability of the Pauli exclusion principle to the chemical bond. Moreover, the quantum-mechanical uncertainty in momentum and coordinate, in fact, strictly indicates that in the chemical bond, electrons are a system of “maximally” strongly interacting particles, and the whole chemical bond is a separate particle in which there is no place for the notion of an “individual” electron, its velocity, coordinate, energy, etc., description. This is fundamentally not true. The chemical bond is a separate particle, called us “semi-virtual particle”, it is a composite particle that consists of individual electrons (strongly interacting), and spatially located between the nuclei.
Thus, the introduction of a three-electron bond with a multiplicity of 1.5 is justified from the chemical point of view (simply explains the structure of the benzene molecule, aromaticity, the structure of organic and inorganic substances, etc.) is confirmed by the Pauli exclusion principle and the logical assumption of a chemical bond as system of strongly interacting particles (actually a separate semi-virtual particle), and as a consequence the inapplicability of the Pauli exclusion principle to a chemical bond.
1. Pauli W. Uber den Zusammenhang des Abschlusses der Elektronengruppen in Atom mit der Komplexstruktur der Spektren, – Z. Phys., 1925, 31, 765-783.
2. A.S. Davydov. Quantum mechanics. Second edition. Publishing house “Science”. Moscow, 1973, p. 334.
Heisenberg’s uncertainty principle and chemical bond.
For further analysis of chemical bond, let us consider the Compton wavelength of an electron:
λc.е. = h/(me*c)= 2.4263 * 10^(-12) m
The Compton wavelength of an electron is equivalent to the wavelength of a photon whose energy is equal to the rest energy of the electron itself (the standard conclusion is given below):
λ = h/(m*v), E = h*γ, E = me*c^2, c = γ*λ, γ = c/λ
E = h*γ, E = h*(c/λ) = me*c^2, λc.е. = h/(me*c)
where λ is the Louis de Broglie wavelength, me is the mass of the electron, c, γ is the speed and frequency of light, and h is the Planck constant.
It is more interesting to consider what happens to an electron in a region with linear dimensions smaller than the Compton wavelength of an electron. According to Heisenberg uncertainty in this area, we have a quantum mechanical uncertainty in the momentum of at least m*c and a quantum mechanical uncertainty in the energy of at least me*c^2 :
Δp ≥ mе*c and ΔE ≥ me*c^2
which is sufficient for the production of virtual electron-positron pairs. Therefore, in such a region the electron can no longer be regarded as a “point object”, since it (an electron) spends part of its time in the state “electron + pair (positron + electron)”. As a result of the above, an electron at distances smaller than the Compton length is a system with an infinite number of degrees of freedom and its interaction should be described within the framework of quantum field theory. Most importantly, the transition to the intermediate state “electron + pair (positron + electron)” carried per time ~ λc.е./c
Δt = λc.е./c = 2.4263 * 10^(-12)/(3*10^8) = 8.1*10^(-20) s
Now we will try to use all the above-mentioned to describe the chemical bond using Einstein’s theory of relativity and Heisenberg’s uncertainty principle. To do this, let’s make one assumption: suppose that the wavelength of an electron on a Bohr orbit (the hydrogen atom) is the same Compton wavelength of an electron, but in another frame of reference, and as a result there is a 137-times greater Compton wavelength (due to the effects of relativity theory):
λc.е. = h/(me*c) = 2.4263 * 10^(-12) m
λb. = h/(me*v)= 2*π*R = 3.31*10^(-10) m
λb./λc.е.= 137
where R= 0.527 Å, the Bohr radius.
Since the De Broglie wavelength in a hydrogen atom (according to Bohr) is 137 times larger than the Compton wavelength of an electron, it is quite logical to assume that the energy interactions will be 137 times weaker (the longer the photon wavelength, the lower the frequency, and hence the energy ). We note that 1 / 137.036 is a fine structure constant, the fundamental physical constant characterizing the force of electromagnetic interaction was introduced into science in 1916 year by the German physicist Arnold Sommerfeld as a measure of relativistic corrections in describing atomic spectra within the framework of the model of the N. Bohr atom.
To describe the chemical bond, we use the Heisenberg uncertainty principle:
Δx * Δp ≥ ћ / 2
Given the weakening of the energy interaction 137 times, the Heisenberg uncertainty principle can be written in the form:
Δx* Δp ≥ (ћ * 137)/2
According to the last equation, the quantum mechanical uncertainty in the momentum of an electron in a chemical bond must be at least me * c, and the quantum mechanical uncertainty in the energy is not less than me * c ^ 2, which should also be sufficient for the production of virtual electron-positron pairs.
Therefore, in the field of chemical bonding, in this case, an electron can not be regarded as a “point object”, since it (an electron) will spend part of its time in the state “electron + pair (positron + electron)”, and therefore its interaction should be described in the framework of quantum field theory.
This approach makes it possible to explain how, in the case of many-electron chemical bonds (two-electron, three-electron, etc.), repulsion between electrons is overcome: since the chemical bond is actually a “boiling mass” of electrons and positrons, virtual positrons “help” overcome the repulsion between electrons. This approach assumes that the chemical bond is in fact a closed spatial bag (a potential well in the energy sense), in which “boiling” of real electrons and also virtual positrons and electrons occurs, and the “volume” of this potential bag is actually a “volume” of chemical bond and also the spatial measure of the quantum-mechanical uncertainty in the position of the electron.
Strictly speaking, with such a consideration, the electron no longer has a certain energy, momentum, coordinates, and is no longer a “point particle”, but actually takes up the “whole volume” of chemical bonding. It can be argued that in the chemical bond a single electron is depersonalized and loses its individuality, in fact it does not exist, but there is a “boiling mass” of real electrons and virtual positrons and electrons that by fluctuate change each other. That is, the chemical bond is actually a separate particle, as already mentioned, a semi-virtual particle. Moreover, this approach can be extended to the structure of elementary particles such as an electron or a positron: an elementary particle in this consideration is a fluctuating vacuum closed in a certain spatial bag, which is a potential well for these fluctuations.
It is especially worth noting that in this consideration, electrons are strongly interacting particles, and therefore the Pauli principle is not applicable to chemical bond (for more details, see the section “The Pauli Principle and the Chemical Bond”) and does not prohibit the existence of the same three-electron bonds with a multiplicity of 1.5.
The above is easy to demonstrate with the example of a chemical bond of 1 Å length. Then the wavelength of Broglie is written in the form (the length of the chemical bond is L = 2 * Δx):
λ = 2*π*Δx
and the Heisenberg uncertainty ratio takes the form:
Δx * Δp ≥ (ћ * 137 * 2 * π) / 2
from which we get:
L * Δp ≥ ћ * 137 * 2 * π
where L is the length of the chemical bond, and Δp is the quantum mechanical uncertainty of the momentum of each electron in a given chemical bond.
Whence, we obtain a formula for determining the uncertainty of the momentum in a chemical bond:
Δp ≥ (ћ * 137 * 2 * π) / L
Having made the necessary calculations for a length of 1 Å, we obtain:
Δp ≥ (ћ * 137 * 2 * π) / 10 ^ (-10)
Δp ≥ 9.078 * 10 ^ (-22) kg * m / s
That is, the uncertainty in the pulse is greater than me * c
(me * c = 2.73 * 10 ^ (-22) kg * m / s)
(it is clear that the uncertainty of the electron velocity will be greater than the speed of light), which should be based on our assumptions.
See pp. 88 – 104 Review. Benzene on the Basis of the Three-Electron Bond. (The Pauli exclusion principle, Heisenberg’s uncertainty principle and chemical bond). http://vixra.org/pdf/1710.0326v1.pdf
Benzene on the basis of the three-electron bond:
Review. Benzene on the Basis of the Three-Electron Bond. (The Pauli exclusion principle, Heisenberg’s uncertainty principle and chemical bond). http://vixra.org/pdf/1710.0326v1.pdf
1. Structure of the benzene molecule on the basis of the three-electron bond.
http://vixra.org/pdf/1606.0152v1.pdf
2. Experimental confirmation of the existence of the three-electron bond and theoretical basis ot its existence.
http://vixra.org/pdf/1606.0151v2.pdf
3. A short analysis of chemical bonds.
http://vixra.org/pdf/1606.0149v2.pdf
4. Supplement to the theoretical justification of existence of the three-electron bond.
http://vixra.org/pdf/1606.0150v2.pdf
5. Theory of three-electrone bond in the four works with brief comments.
http://vixra.org/pdf/1607.0022v2.pdf
6. REVIEW. Benzene on the basis of the three-electron bond. http://vixra.org/pdf/1612.0018v5.pdf
7. Quantum-mechanical aspects of the L. Pauling’s resonance theory.
http://vixra.org/pdf/1702.0333v2.pdf
8. Quantum-mechanical analysis of the MO method and VB method from the position of PQS.
http://vixra.org/pdf/1704.0068v1.pdf
9. Review. Benzene on the Basis of the Three-Electron Bond. (The Pauli exclusion principle, Heisenberg’s uncertainty principle and chemical bond). http://vixra.org/pdf/1710.0326v1.pdf
Bezverkhniy Volodymyr (viXra):http://vixra.org/author/bezverkhniy_volodymyr_dmytrovych
Thank you James (and also Matt) for another really illustrative post.
Another thing that has always bugged me, which is also related to this topic, is the hybridization of Oxygen (especially explaining the bond angle of 104.5° for water).
I have spoken to a lot of people and professors and it seems that the opinions on this are pretty much split.
On the one hand, there is people saying that Oxygen hybridizes just as Carbon does and that the two lone pairs push the angle from 109.5° to 104.5°.
On the other hand, people claim that the s and p-orbitals of Oxygen are energetically too far apart to hybridize and this model is just an easy but inaccurate explanation.
According to this, the two Hydrogens bond to two p-orbitals at an angle of 90°, but due to repulsion don’t sit exactly on the p-axes but are slightly off (104.5°), resulting in a poorer orbital overlap (unfavorable, but more favorable than hybridization).
A third approach i have heard is bent’s rule (Henry bent), which says that in an sp^n hybrid-orbital, n does not have to be a whole integer. So the orbitals for the lone pairs would have more s-character (because closer to the nucleus) and for the Hydrogens more p-character (because further away from nucleus).
Maybe some day, if you have the time and resources you could discuss this topic.
I would really be interested in your take on this.
Best regards from Austria.